What is " waterfall smearing"?

When analysing a waterfall or performing order analysis it is important to consider the frequency resolution or the frequency spacing.

There is often a desire to increase the resolution to finer and finer detail. But that is a process of diminishing returns, and actually fraught with danger. And that danger is waterfall smearing.

Figure-1 shows a waterfall created with a suitable frequency resolution. This has very little or no smearing.

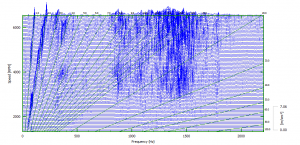
Figure 1: Waterfall created using a suitable frequency resolution

To increase the resolution further, the block size has to become larger, the block sizes are always a power of 2.

The danger is the bigger the block size the more smearing in the waterfall. Waterfall smearing is caused by a wide range of speeds being included in each block.

The waterfall plot is speed based. If the speed is changing quickly, say 100RPM per second and we have chosen a block size that encompasses 4 seconds of data then the RPM will have changed by 400RPM in that time. The orders will appear to ‘smear’ along the speed axis. This is waterfall smearing. See the example in Figure 2.

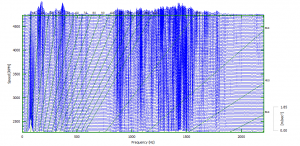
Figure 2: The same data as Figure 1 analysed with a bigger block size

Figure 2 shows the same data as figure 1, but with a larger FFT block size.

In figure 1 the 2^nd order is clearly visible, increasing with speed, but in figure 2 that same 2^nd order is very unclear. The changes in speed have meant that the block size is too large and thus the speed has changed inside the FFT block. Further each FFT block is overlapping with the previous and the next.

The result is a waterfall or order analysis that is smeared.

Bio
Latest Posts

James Wren

Former Sales & Marketing Manager at Prosig

James Wren was Sales & Marketing Manager for Prosig Ltd until 2019. James graduated from Portsmouth University in 2001, with a Masters degree in Electronic Engineering. He is a Chartered Engineer and a registered Eur Ing. He has been involved with motorsport from a very early age with a special interest in data acquisition. James is a founder member of the Dalmeny Racing team.